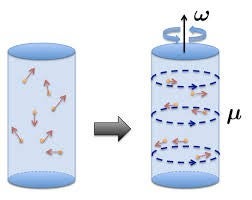
This image depicts a concept from physics involving the interaction between a magnetic field and a conductive material within the context of resonance and rotational motion. Here’s an interpretation of what the image suggests:
- Left Diagram: A cylindrical bar, possibly iron due to its magnetic properties, is shown with arrows inside it. These arrows could represent magnetic domains or moments within the iron bar that are randomly oriented, implying the bar is not magnetized.
- Right Diagram: The same bar is now rotating, as indicated by the angular velocity vector (ω) at the top. This rotation is causing the magnetic moments to align due to the phenomenon known as the Einstein-de Haas effect, which relates the angular momentum of the bar with the magnetic moment (µ). The rotation induces a magnetic field, aligning the domains.
- Copper Coil: The coil surrounding the bar, colored in shades of orange and cyan, represents parts of a copper coil that are in different positions relative to the movement of the bar's magnetic field. Orange parts are near the node, where there is minimal movement of the bar, and the cyan parts are near the antinode, where the movement is greatest in a standing wave pattern.
- Resonance: If the bar rotation speed matches the resonant frequency of some standing wave pattern within the bar, then resonance can occur. This resonance can amplify the effect of the rotating magnetic field on the copper coil.
- Induced Current: The interaction between the rotating magnetic field of the iron bar and the copper coil would induce an electrical current in the coil according to Faraday's law of electromagnetic induction.
In an application like an electric generator, this principle is utilized to convert mechanical energy into electrical energy. The depiction simplifies the process, omitting many real-world complexities, but captures the essence of how magnetic fields and motion can induce electrical currents.

April 21, 2024

