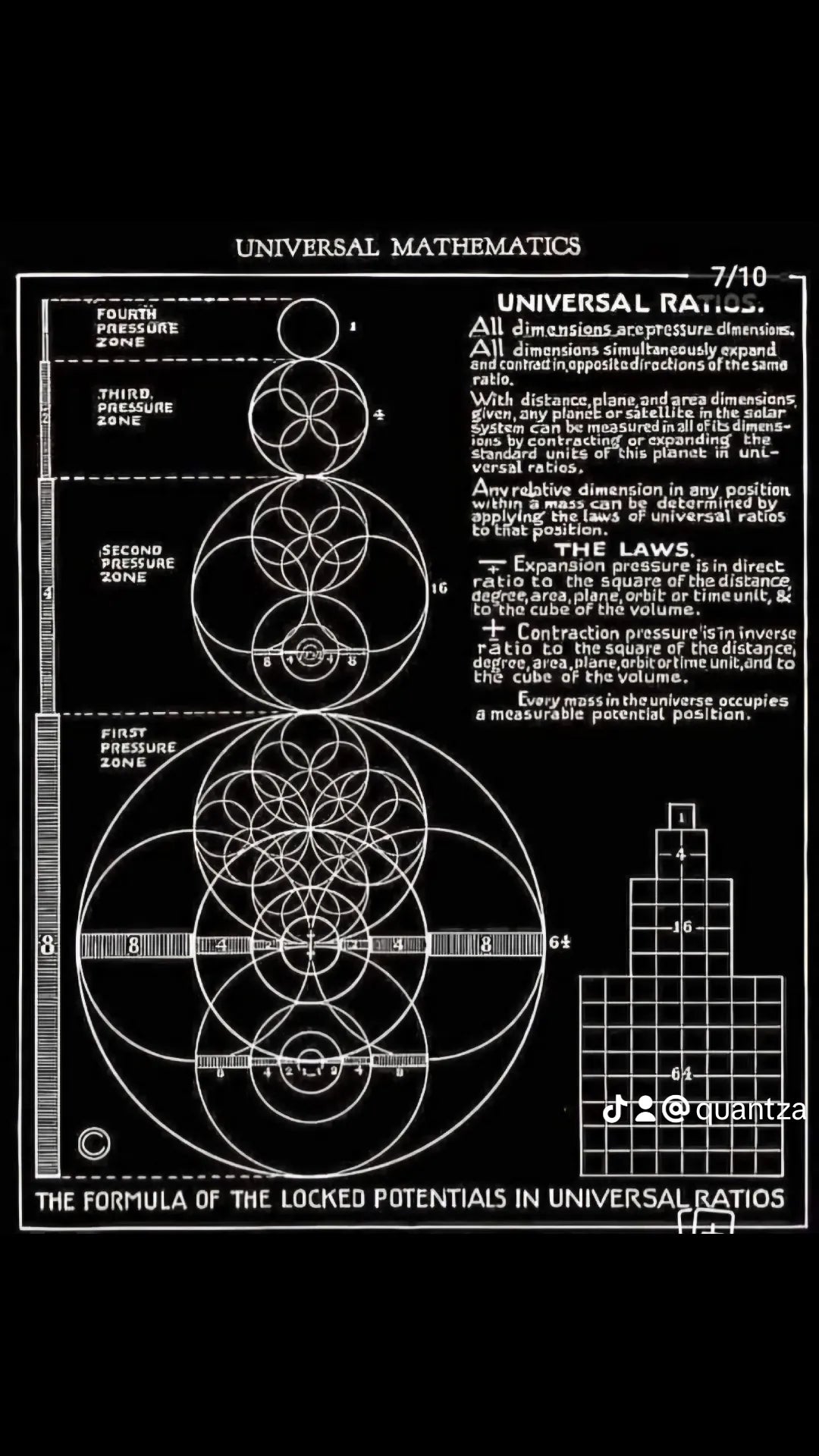
The image you provided is a diagram related to "Universal Mathematics" and "Universal Ratios." Here’s an explanation of its main components:
#UniversalRatios
- **Dimensions as Pressure Dimensions**: The diagram suggests that all dimensions are pressure dimensions and they expand and contract simultaneously in opposite directions of the same ratio.
- **Measurement Using Universal Ratios**: It implies that distances, planes, and area dimensions of any object (such as a planet or satellite in the solar system) can be measured by contracting or expanding standard units using universal ratios.
- **Relative Dimensions**: The idea is that any dimension in any position within a mass can be determined by applying these universal ratios.
#TheLaws
1. **Expansion Pressure**:
- Expansion pressure is directly proportional to the square of the distance (or degree, area, plane, orbit or time unit) and to the cube of the volume.
2. **Contraction Pressure**:
- Contraction pressure is inversely proportional to the square of the distance (or degree, area, plane, orbit or time unit) and to the cube of the volume.
#Diagram #Components
- **Pressure Zones**: There are four pressure zones illustrated, each presumably representing different scales or levels of pressure:
- Fourth Pressure Zone
- Third Pressure Zone
- Second Pressure Zone
- First Pressure Zone
- **Geometric Patterns**: The overlapping circles and other geometric shapes might represent different fields of pressure or dimensions interacting with each other.
- **Measurable Potential Position**: Every mass in the universe occupies a measurable potential position according to the principles illustrated.
- **The Formula**: The bottom section mentions "The Formula of the Locked Potentials in Universal Ratios," suggesting a formulaic approach to understanding how these pressures and dimensions interact.
#Grids and #Numbers
- **Grids**: The grids with numbers (1, 4, 16, etc.) might represent quantifiable units of measure for these dimensions or pressure zones.
This diagram represents a complex theoretical framework where dimensions and pressures are interlinked through universal ratios. It implies that everything in the universe has a measurable position based on these ratios, expanding and contracting in predictable ways. The laws provided give mathematical relationships for understanding these dynamics.
To delve deeper into the patterns of universal ratios and how they expand according to the diagram, we can break down the components and laws provided. Here's a more detailed analysis:
#Patterns of #Universal #Ratios
1. Expansion and Contraction in Opposite Directions
The universal ratios indicate that all dimensions expand and contract simultaneously but in opposite directions. This could be visualized through geometric shapes such as circles and spheres expanding outward while their counterparts contract inward.
2. Pressure Zones
There are four distinct pressure zones represented:
- **First Pressure Zone**: Base level with fundamental ratios.
- **Second Pressure Zone**: Higher complexity and larger scales.
- **Third Pressure Zone**: Even more complex interactions and larger scales.
- **Fourth Pressure Zone**: Highest level, possibly encompassing the entire system or universe.
Expansion Pressure (Direct Proportionality)
According to the diagram, expansion pressure follows these rules:
- **Square of Distance, Degree, Area, Plane, Orbit, or Time Unit**: If \( d \) is the distance, then expansion pressure \( P_e \) is proportional to \( d^2 \).
- **Cube of Volume**: If \( V \) is the volume, then expansion pressure is proportional to \( V^3 \).
Mathematically, this could be represented as:
\[ P_e \propto d^2 \]
\[ P_e \propto V^3 \]
#Contraction #Pressure (Inverse Proportionality)
For contraction pressure:
- **Square of Distance, Degree, Area, Plane, Orbit, or Time Unit**: If \( d \) is the distance, then contraction pressure \( P_c \) is inversely proportional to \( d^2 \).
- **Cube of Volume**: If \( V \) is the volume, then contraction pressure is inversely proportional to \( V^3 \).
#Mathematically:
\[ P_c \propto \frac{1}{d^2} \]
\[ P_c \propto \frac{1}{V^3} \]
### Geometric Patterns
- **Circles and Spheres**: The diagram shows nested and overlapping circles, possibly representing different pressure zones or scales. The radii of these circles could be expanding and contracting based on the principles mentioned.
- **Interlocking Circles**: These might symbolize how different dimensions or zones interact, with each smaller circle fitting into the larger ones, showing a fractal-like pattern of expansion and contraction.
### Grids and Numerical Patterns
- **Numerical Grid**: The grid with numbers 1, 4, 16, etc., could indicate scales or units of measure for these dimensions and pressures. These numbers suggest a pattern where each number is a power of 2 (e.g., \( 2^0 = 1 \), \( 2^2 = 4 \), \( 2^4 = 16 \), etc.).
#Practical #Implications
- **Measuring Universal Properties**: Using these ratios, one could theoretically measure properties like mass, volume, and distance in universal terms.
- **Predictive Models**: These patterns could help in creating models to predict how objects in the universe expand or contract under different pressures.
### Summary
The universal ratios and pressure zones provide a framework for understanding the expansion and contraction of dimensions in the universe. The key patterns involve direct and inverse proportionality to the square of distances and the cube of volumes, represented through geometric and numerical patterns. These concepts can be applied to measure and predict universal properties and dynamics.

May 25, 2024

